
Two angles are supplementary and have a ratio of 1:4. What is the size of the smaller angle?
Possible Answers: Correct answer: Explanation :Since the angles are supplementary, their sum is 180 degrees. Because they are in a ratio of 1:4, the following expression could be written:
AB and CD are two parrellel lines intersected by line EF. If the measure of angle 1 is , what is the measure of angle 2?
Possible Answers: Correct answer: Explanation :The angles are equal. When two parallel lines are intersected by a transversal, the corresponding angles have the same measure.
Figure not drawn to scale.
In the figure above, APB forms a straight line. If the measure of angle APC is eighty-one degrees larger than the measure of angle DPB, and the measures of angles CPD and DPB are equal, then what is the measure, in degrees, of angle CPB?
Possible Answers: Correct answer: Explanation :Let x equal the measure of angle DPB. Because the measure of angle APC is eighty-one degrees larger than the measure of DPB, we can represent this angle's measure as x + 81. Also, because the measure of angle CPD is equal to the measure of angle DPB, we can represent the measure of CPD as x.
Since APB is a straight line, the sum of the measures of angles DPB, APC, and CPD must all equal 180; therefore, we can write the following equation to find x:
x + (x + 81) + x = 180
Simplify by collecting the x terms.
Subtract 81 from both sides.
This means that the measures of angles DPB and CPD are both equal to 33 degrees. The original question asks us to find the measure of angle CPB, which is equal to the sum of the measures of angles DPB and CPD.
measure of CPB = 33 + 33 = 66.
The answer is 66.
One-half of the measure of the supplement of angle ABC is equal to the twice the measure of angle ABC. What is the measure, in degrees, of the complement of angle ABC?
Possible Answers: Correct answer: Explanation :Let x equal the measure of angle ABC, let y equal the measure of the supplement of angle ABC, and let z equal the measure of the complement of angle ABC.
Because x and y are supplements, the sum of their measures must equal 180. In other words, x + y = 180.
We are told that one-half of the measure of the supplement is equal to twice the measure of ABC. We could write this equation as follows:
Because x + y = 180, we can solve for y in terms of x by subtracting x from both sides. In other words, y = 180 – x. Next, we can substitute this value into the equation (1/2)y = 2x and then solve for x.
Multiply both sides by 2 to get rid of the fraction.
Add x to both sides.
Divide both sides by 5.
The measure of angle ABC is 36 degrees. However, the original question asks us to find the measure of the complement of ABC, which we denoted previously as z. Because the sum of the measure of an angle and the measure of its complement equals 90, we can write the following equation:
Now, we can substitute 36 as the value of x and then solve for z.
Subtract 36 from both sides.
The answer is 54.

In the diagram, AB || CD. What is the value of a+b?
Possible Answers:None of the other answers.
Correct answer: Explanation :Refer to the following diagram while reading the explanation:

We know that angle b has to be equal to its vertical angle (the angle directly "across" the intersection). Therefore, it is 20°.
Furthermore, given the properties of parallel lines, we know that the supplementary angle to a must be 40°. Based on the rule for supplements, we know that a + 40° = 180°. Solving for a, we get a = 140°.
Therefore, a + b = 140° + 20° = 160°
In rectangle ABCD, both diagonals are drawn and intersect at point E.
Let the measure of angle AEB equal x degrees.
Let the measure of angle BEC equal y degrees.
Let the measure of angle CED equal z degrees.
Find the measure of angle AED in terms of x, y, and/or z.
Possible Answers: Correct answer: Explanation :Intersecting lines create two pairs of vertical angles which are congruent. Therefore, we can deduce that y = measure of angle AED.
Furthermore, intersecting lines create adjacent angles that are supplementary (sum to 180 degrees). Therefore, we can deduce that x + y + z + (measure of angle AED) = 360.
Substituting the first equation into the second equation, we get
x + (measure of angle AED) + z + (measure of angle AED) = 360
2(measure of angle AED) + x + z = 360
2(measure of angle AED) = 360 – (x + z)
Divide by two and get:
measure of angle AED = 180 – 1/2(x + z)
A student creates a challenge for his friend. He first draws a square, the adds the line for each of the 2 diagonals. Finally, he asks his friend to draw the circle that has the most intersections possible.
How many intersections will this circle have?
Possible Answers: Correct answer: Explanation : The answer to this problem is 12. This can be drawn as shown below (intersections marked in red).
We can also be sure that this is the maximal case because it is the largest answer selection. Were it not given as a multiple choice question, however, we could still be sure this was the largest. This is because no line can intersect a circle in more than 2 points. Keeping this in mind, we look at the construction of our initial shape. The square has 4 lines, and then each diagonal is an additional 2. We have thus drawn in 6 lines. The maximum number of intersections is therefore going to be twice this, or 12.
Two pairs of parallel lines intersect:

If A = 135 o , what is 2*|B-C| = ?
Possible Answers: Correct answer: Explanation :By properties of parallel lines A+B = 180 o , B = 45 o , C = A = 135 o , so 2*|B-C| = 2* |45-135| = 180 o
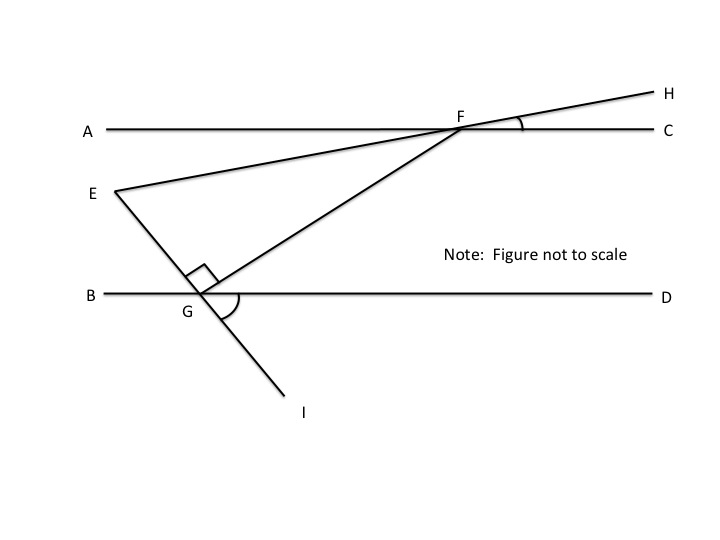
Lines and are parallel. , , is a right triangle, and has a length of 10. What is the length of
Possible Answers:Not enough information.
Correct answer: Explanation :Since we know opposite angles are equal, it follows that angle and .
Imagine a parallel line passing through point . The imaginary line would make opposite angles with & , the sum of which would equal . Therefore, .
If measures , which of the following is equivalent to the measure of the supplement of ?
Possible Answers: Correct answer: Explanation :When the measure of an angle is added to the measure of its supplement, the result is always 180 degrees. Put differently, two angles are said to be supplementary if the sum of their measures is 180 degrees. For example, two angles whose measures are 50 degrees and 130 degrees are supplementary, because the sum of 50 and 130 degrees is 180 degrees. We can thus write the following equation:
Subtract 40 from both sides.
Add to both sides.
← Previous 1 2 3 4 5 6 7 8 9 … 79 80 Next →
Dartmouth College, Bachelor in Arts, Biochemistry and Molecular Biology. Columbia University in the City of New York, Master .


Cleveland State University, Bachelor of Science, Mathematics. University of Portland, Masters in Education, Curriculum and In.
If you've found an issue with this question, please let us know. With the help of the community we can continue to improve our educational resources.
If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.
Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.
Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.
Please follow these steps to file a notice:
You must include the following:
A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.
Send your complaint to our designated agent at:
Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105
Or fill out the form below: